A geometric realization of hyperbolic Coxeter groups

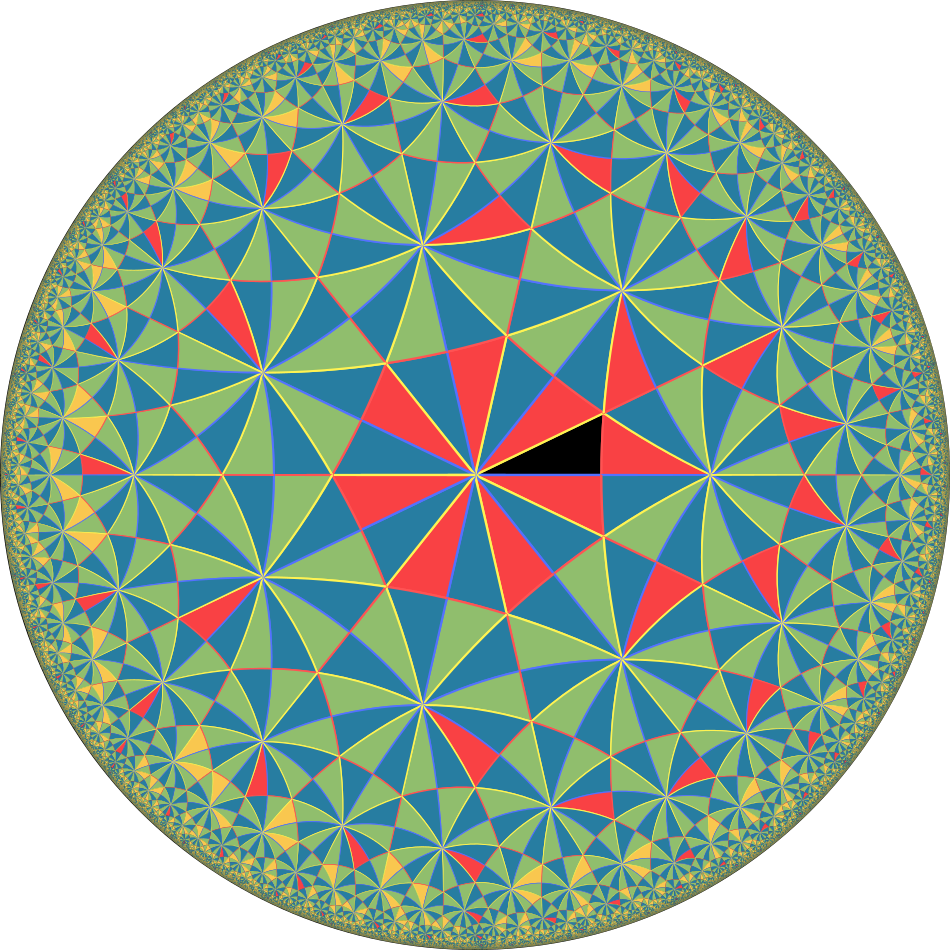
In the picture above, you can see a geometric realisation of the Coxeter group $W$ with presentation

\left\langle s_1, s_2, s_3 ~\middle|~ (s_1s_2)^2 = (s_2s_3)^3 = (s_3s_1)^7 = s_1^2 = s_2^2 = s_3^2 = e \right\rangle

as a reflection group acting on the hyperbolic plane in the Poincaré ball model. Different colored segments of the hyperplanes correspond to multiplication with different generators from the right. There is a bijection between elements in $W$ and tiles. The color of a tile indicates the reflection length of the associated group element, where the identity chamber is black.

This picture was created during the DAAD funded RISE Germany internship "Programming a geometric realization of hyperbolic Coxeter groups'' by Mireia Taus and Marco Lotz.

For more details and visuals, Marco Lotz explains this project along with the mathematics of hyperbolic space in a short presentation for the Heidelberg Experimental Geometry Lab here: